1. Evaluate the determinant of the matrix

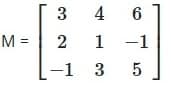
• A. 20
• B. 25
• C. 30
• D. 35

2. Find the amount if simple interest is paid yearly at 5% per annum for 3 years, on a principal of # 200,000.00

• A. # 23, 000.00
• B. # 30,000.00
• C. # 300,000.00
• D. # 230,000.00

3. y is inversely proportional to x and y = 4, when x = 1212, find x when y = 10

• A. 2
• B. 10
• C. 1/5
• D. 1/10

4. Find the angle subtended by an arc 33cm long of a circle of diameter 28cm.

• A. 105⁰
• B. 115⁰
• C. 120⁰
• D. 135⁰

5. Solve 16xx = 0.25

• A. -1/2
• B. 1/4
• C. -1/4
• D. 1/2

6. The ratio of men to women in a 20-member committee is 3:1. How many women must be added to the committee to make the ratio of men to women 3:2?

• A. 2
• B. 5
• C. 7
• D. 8

7. If p * q = 2p + pq + q, find p  when ( p * 2) – (p * 1) = 40

• A. 39
• B. 40
• C. 41
• D. 42

8. Which of the following is a measure of central tendency?

• A. Standard deviation
• B. Range
• C. Variance
• D. Mean

9.

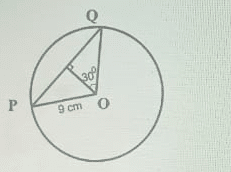
From the figure above, find the length of Chord PQ

• A. 17cm
• B. 18cm
• C. 7cm
• D. 9cm

10.

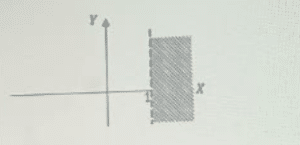
Identify the equation of the shaded region in the given graph 

• A. y > 1
• B. x < 1
• C. X > 1
• D. y < 1

11. In how many ways can 6 people sit around a table 

• A. 120
• B. 240
• C. 60
• D. 30

12.

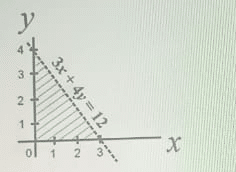
The graph above represents inequalities

• A. 3x +4y ≥12, x >0, y > 0
• B. 3x + 4y≤ 12, x ≥ 0, y ≥ 0
• C. 3x + 4y <12, x ≤ 0, y ≥ 0
• D. 3x + 4y < 12, x ≥ 0, y ≥ 0

13.

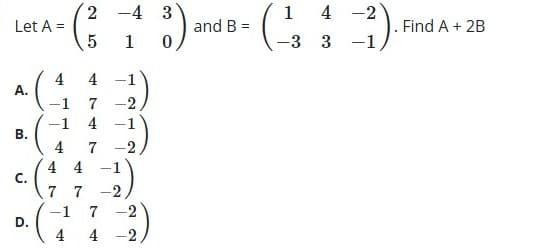
14. The sum to infinity of a GP is 100, find its first term if the common ratio is -1212

• A. 150
• B. 155
• C. 160
• D. 165

15. PQR is a triangle such that |PQ| = |QR| = 8cm and QPR = 60º. Find the area of ∠∠PQR

• A. 16√(2) cm²
• B. 16√(2) cm²
• C. 8√(3)cm²
• D. 16√(2)cm²

16.

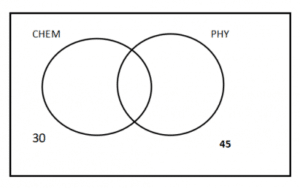
The Venn diagram above shows the number of students offering physics and chemistry in a class of 65. What is the probability that a student selected from the class offers physics and chemistry if every students offers at least one subject?

• A. 2/13
• B. 5/13
• C. 2/5
• D. 3/13

17. From the top of a building 10m high, the angle of elevation of a fruit on top of a tree 25m is 30º. Calculate the horizontal distance between the building and the tree.

• A. 15√3m
• B. 5√3m
• C. 25√3m
• D. 30√3m

18. A boy bought Oranges at the rate of #24.00 for 5 and sold it at the rate of # 30.00 for 4 Oranges. Find the profit made of the ones sold 

• A. # 24.00
• B. #30.00
• C. # 10.80
• D. #20.00

19. Differentiate y = (5x + 1)⁴

• A. 20 (5x + 1)³
• B. 4(5x + 1)³
• C. 5(5x + 1)³
• D. (5x)³

20. Simplify (5+√7)/(3+√7)

• A. 17 – √7
• B. 4 – √7
• C. 7 – √7
• D. 15 – √7

21. Find the perimeter of a triangle whose vertices pass through ( 3, 2), (4, 5) and (6, 2) in surd form.

• A. 3 + √12+√13
• B. 3 – √10 + √13
• C. 3 + √10 + √13
• D. 3 – √12 + √13

22. Find the 7th term of the sequence -10, 50, -250 ………..

• A. 165250
• B. 156250
• C. -156250
• D. -165,250

23. The scores of students in a test are recorded as follows: 4, 3, 3, 2, 1, 2, 5, 7, 8, 3, and 5. Find the mode of the mark.

• A. 2
• B. 3
• C. 4
• D. 5

24. P(x, 4) and Q( 10, 8) are two points joined by a straight line in a plane. If the midpoint of the line is (9, 6), find the value of x.

• A. 12
• B. 9
• C. 8
• D. 7

25. Find x if  = (16^2+x)/4 = 64^x

• A. 3
• B. – 3
• C. 5
• D. – 5

26. Given that P is the set of all prime numbers between 0 and 10, and Q is the set of all odd numbers between 0 and 10. Find the union of elements in P that are not in Q and the elements in Q that are not in P.

• A. {2, 9}
• B. { 9}
• C. {1, 9}
• D. {1, 2, 9}

27. 

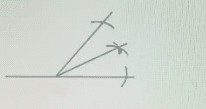
In the diagram above, T represents the construction of angle …..

• A. 15⁰
• B. 30⁰
• C. 45⁰
• D. 60⁰

28. Convert the number 10111.11_twoto a mixed number.

• A. 23 (1/4)
• B. 23 (3/4)
• C. 22 (1/4)
• D. 24 (3/4)

29. Subtract 14256_sevenfrom 20045_seven

• A. 1256_seven
• B. 2456_seven
• C. 1356_seven
• D. 2465_seven

30. The average age of the four female teachers in a school is 40 and the average age of eight male teachers in the school is 25. Calculate the average age of the teachers in the school.

• A. 20
• B. 31
• C. 32
• D. 30

31. How many proper and improper subsets are there in the set K = { a, b, c, d, e}?

• A. 10
• B. 25
• C. 32
• D. 50

32. Differentiate Cos25⁰ – Sin 25⁰

 

• A. – ( Sin25⁰ + Cos25⁰)  
• B. – ( Sec25 + Cot25)  
• C. – ( Cot25 + Sec25)  
• D. – ( Sin25 + Sec25)  

33. The mean of the numbers 13, 16, x, 18, 21, 2x, 35, is 22. Find the value of x

• A. 16
• B. 17
• C. 23
• D. 25

34. Find the variance of a group of data whose standard deviation is 12.34 to the nearest whole number.

• A. 150
• B. 151
• C. 152
• D. 153

35. Calculate the standard deviation of the following scores 5, 4, 6, 7, and 8

• A. √2
• B. √3
• C. 2
• D. 3

36. From a class of 5 girls and 7 boys, a committee consisting of 2 girls and 3 boys is to be formed. How many ways can this be done?

• A. 350 ways
• B. 420 ways
• C. 550 ways
• D. 720 ways

37. In how many ways can a committee of 5 be selected from a group of 7 males and 3 females, if the committee must have one female?

• A. 105ways
• B. 135 ways
• C. 210 ways
• D. 231 ways

38. A bag contains 7 red and 4 black identical balls. Two balls were picked at random from the bag and replaced each time. Find the probability the two balls were of same colour.

• A. 54/121
• B. 65/121
• C. 29/55
• D. 27/55

39. U varies directly as the square root of V when U = 24, V = 9, find the value of V when U = 16.

• A. 0.32
• B. 4
• C. 6
• D. 0.25

40. If B varies inversely as c^(1/3) and C = 27 when B = 2, find the value of the constant of proportionality K.

• A. 3
• B. 6
• C. 9
• D. 12

41. Find the roots of x³– 19x – 30=0

• A. -5, 3, and 2
• B. 5, 3, and -2
• C. 5, -3 and -2
• D. -5, -3 and 2

42. If x is inversely proportional to y and x = 9 when y = 4, find the law containing x and y

• A. x = 16/y
• B. x = 36/y
• C. x = y/36
• D. x = 13/y

 

43.

44.

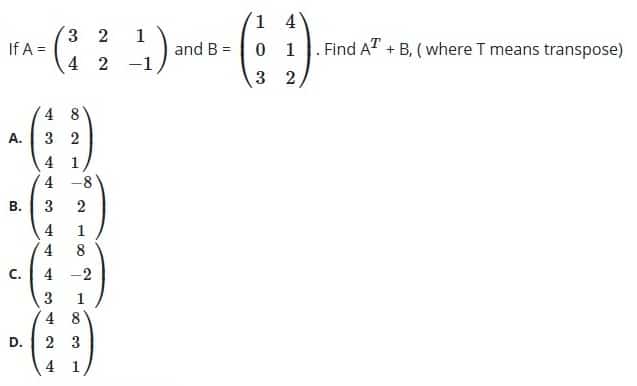
45. If tan θ = 8/15, simplify (Sinθ−Cosθ) / (Sin2θ−Sinθ)

• A. −119/72
• B. 119/72
• C. −7/17
• D. 8/17

46. A binary operation * is defined on a set of real numbers by x*y = x^yfor all values of x and y, if x * 2 = x, find the possible values of x.

• A. 0, 2
• B. 1, 2
• C. 0, 1
• D. 2, 2

47. The weights of 15 students in a class are given as 25, 30, 32, 30, 42, 45, 48, 50, 52, 51, 42, 38, 40, and 42. What is the mode of the given data?

• A. 51
• B. 45
• C. 42
• D. 25

48.

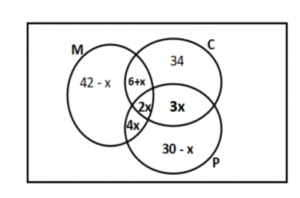
The number of 144 students who registered for mathematics, physics, and chemistry in an examination are shown in the Venn diagram. How many registered for physics and mathematics?

• A. 16
• B. 24
• C. 62
• D. 4

49.

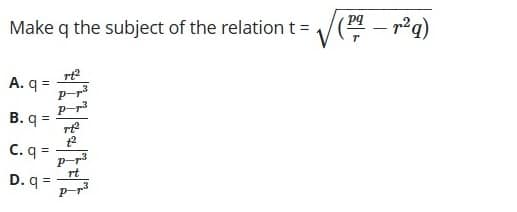
50.

(√a + √(8a))² = 54 + b√2, a and b are positive integers. Find the value of a and the value of b.

• A. a = 24, b = 6
• B. a = 6, b = 2
• C. a = 6, b = 24
• D. a = 2, b = 6